This is an archival copy of the Visualization Group's web page 1998 to 2017. For current information, please vist our group's new web page.

Visualization of Lattice Gauge QCD Simulations at NERSC

The following technical information was copied from Greg Kilcup's Lattice Gauge Theory web page at Ohio State University:

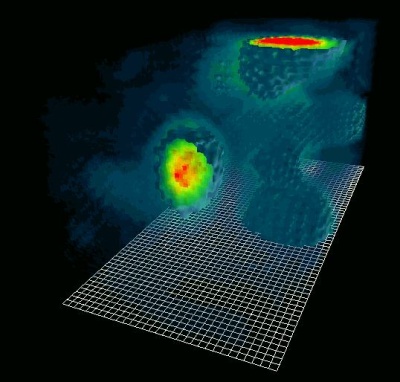
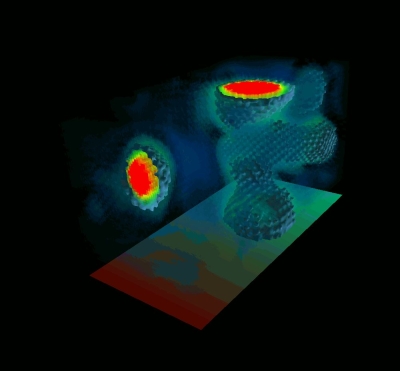
This is a visualization of some of the first data to come off the new 128-node Cray T3E recently installed at NERSC (circa 1997). The still frames below show part of an eigenmode of the Dirac operator. Since we work in four dimensions the full structure cannot be shown in one picture; these images show single three dimensional slices. If your browser is Java-capable, click on one of the images below to animate the frames and see the full four-dimensional object as we loop over the supressed dimension.

Lattice QCD is the subfield of particle theory which attempts to solve QCD problems by approximating spacetime with a discrete grid. Once they are put on a grid, these problems can be attacked by a variety of means, most often involving numerical methods on (super-)computers. The approach was invented by OSU's own Ken Wilson in 1974, and has since evolved in to major discipline within particle physics. The OSU group is currently involved with several large-scale numerical simulations aimed at understanding strong and weak interaction properties of heavy and light quarks. Among these is the collaboration which has recently (Feb 1997) been named one of the Department of Energy Grand Challenges.

We use the simple Wilson plaquette action on a 32*32*32*64 lattice, with beta=6.4, and staggered quarks. The boundaries are periodic in each of the four dimensions. The configuration was cooled slightly (20 steps) to erase short-distance fluctuations. We used inverse iteration to converge to the eigenvector with the smallest eigenvalue. More precisely, we have isolated an eigenvector of Dslash-squared. It is a linear combination of the pair of related lowest eigenvectors of Dslash (with eigenvalues lambda and -lambda). The fact that the eigenmode is localized is interesting. (Higher modes would look like uniform uninteresting planewaves.) Though we have not yet checked explicitly, we expect that the eigenmode is "pinned" on top of instantons, topologically nontrivial knots in the underlying gauge field. These localized modes are a special feature of non-Abelian gauge theories, and are part of what makes QCD so hard to solve. Ultimately, the size and shape of such eigenmodes is believed to be responsible for basic properties of matter, such as the the size and mass of the proton.